My answer: Fuck this just give me a rifle and point me towards the enemy lines!
Fake. All soviets did were live in huts, shoot 17th century rifles, and failed basic economics
welcome to my hobby. collecting and saving out of print communist literature in a hopes of digitizing them and making them immortal on the internet.
I like to think that out of all the ways I could emulate Stalin, this one's the best way to honor his memory.
Do you have a rig for book digitizing? I always had an itch to build one when I was in school but I never did
No, I find books and have them sent to my crew to be digitized then archived into our internal library. I know a few other folks in my crew also do similar things with all sorts of media, ranging from old americanized soviet films on those old fashioned film reels to childrens books and magazines to old music composed by the more esoteric parts of communist history.
I think some of the most recent stuff I was a part of in NY was helping one of my crew's digitizing tech specialists in sorting through old Jewish communist literature and pamphlets, Misha magazines, and old communist vinyl record disks and tape cassettes that CPUSA threw away during their liquidation.
very cool! I am thinking about getting a cnc plywood cutter and designing a flat packable ocr rig would be fun
Just looking up images of a cnc plywood cutter gives my inner creator nerd warm fuzzies just looking at the neat shit you can make with it
I think if I had one I'd try my hand at making a marble racing contraption doohicky and make it as wild as I could make it
I could very easily lose myself in making marble racetracks
you gonna send these over to gen lib or something? a little interested in this one
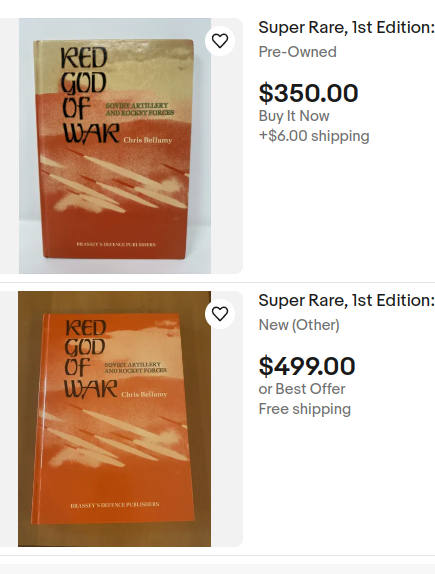
be nice to that thing, I can't find it for less than that ebay screenshot
I feel like if I actually went through the Soviet education system I might be able to do this but my capitalist education was so lacking that I wouldn't even know where to start
One of my math professors grew up in soviet Romania and sometimes told us about what math they were taught when.
He said they were taught some relatively high up stuff (by American math standards) in high school. Some reasonably serious group theory apparently
But I was told I'd be working in the coal mines after the revolution???
yes
I haven't done this type of math in almost 10 years but yes, I'll git gud in time
I am literally training to be a scientist and I fucking can't do math very well at all.
Hahaha the western standard is so low
Trying out the problems to see how my Indian education(Kerala state govt college education) helps me:
-
In the denominator there is a common a on both sides and can be cancelled. In the right hand side there's a common c which can be simplified.
And the numerator on the left can be multiplied by 2/2
We can write 4a2(b+c)2n as (2a(b+c)n)2
Then using x2 - y2 = (x - y) (x + y) we can simplify the numerator. -
3 > ln(x)
Edit: ln(x) is bounded on both sides here. Thanking u/ AernaLingus for pointing that out
The complete answer would be 1/2 < ln(x) < 3
Thanking them again.
-
x = e^(-3 ln(3)/(30 - ln(3)^)
-
What's tg?
Is it tangent? Assumed that and got x = cos-1(1/3) = 1.23095
Well we found our first officer of the artillery corps, everyone.
And the numerator on the left can be multiplied by 2/2
I don't understand this step. Why did you multiply is for 2/2, I don't see how it simplifies the numerator on the left, when expanding the fraction sum leads to 4a(b+c)^2n-1, something simpler to factor using x2-y2=(x+y) (x-y)
(2a2 (b+c)2n - 1/2) x 2/2 = (4a2 (b+c)2n - 1) x 1/2
Let's keep the 1/2 aside for now.We can write 4a2 (b+c)2n as 22 a2 ((b+c)n )2 and 1 as 12
So (4a2 (b+c)2n - 1) = ( 2a(b+c)n )2 - 12
Comparing with x2 - y2 we have x = 2a(b+c)n and y = 1
With with x2 - y2 =(x+y) (x-y), we get:(2a(b+c)n)2 - 12 = (2a(b+c)n + 1) (2a(b+c)n - 1)
We can then cancel the common (2a(b+c)n - 1) term from the numerators on both sides...
with the assumption that 2a(b+c)n - 1 ≠ 0. If it's zero, then we introduce the issue of 0 x something = 0 x something.
0 x 1 = 0 x 2 = 0
We can't cancel the common zero and say that 1 = 2. So 0/0 or 1/0 is an issue, but with the assumption that it isn't 0, we can cancel it out.I multiplied and dividing by 2 because in effect it changes nothing to the result as 2/2 = 1. But it helps to rearrange and simplify stuff.
Could you give me a hand with (2) and (3)? You don't need to work the whole thing out, but a nudge in the right direction would be greatly appreciated. I feel like I mostly have the knowledge to solve these but I'm just forgetting some crucial rule or I'm just too rusty with my algebra (it's been a loooong time since I had to do this stuff).
Here's my work for (2):
Show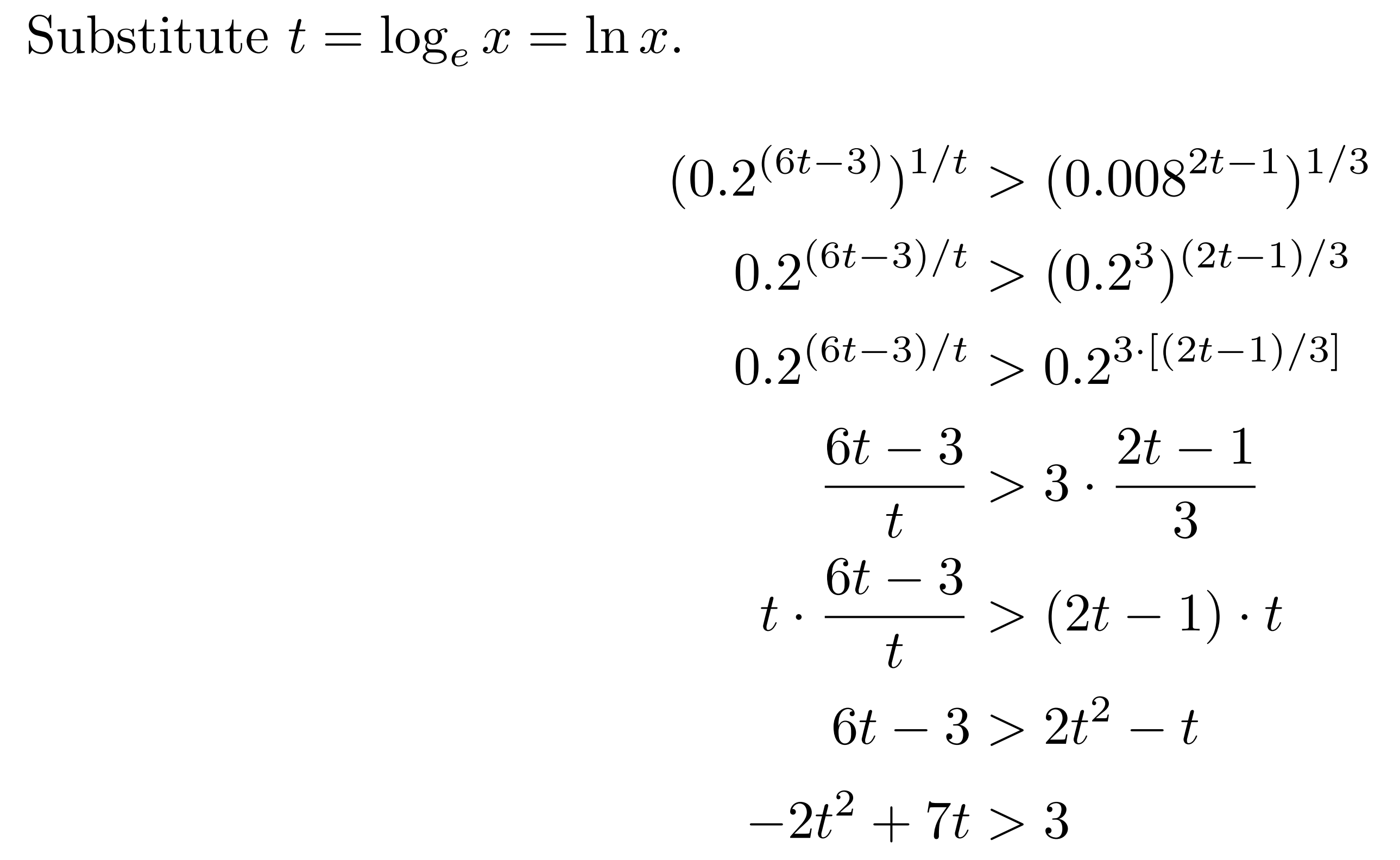
The one part I feel confident about is changing the bases to match so I can just work with the exponents, but the rest feels off.
Here's my work for (3):
Show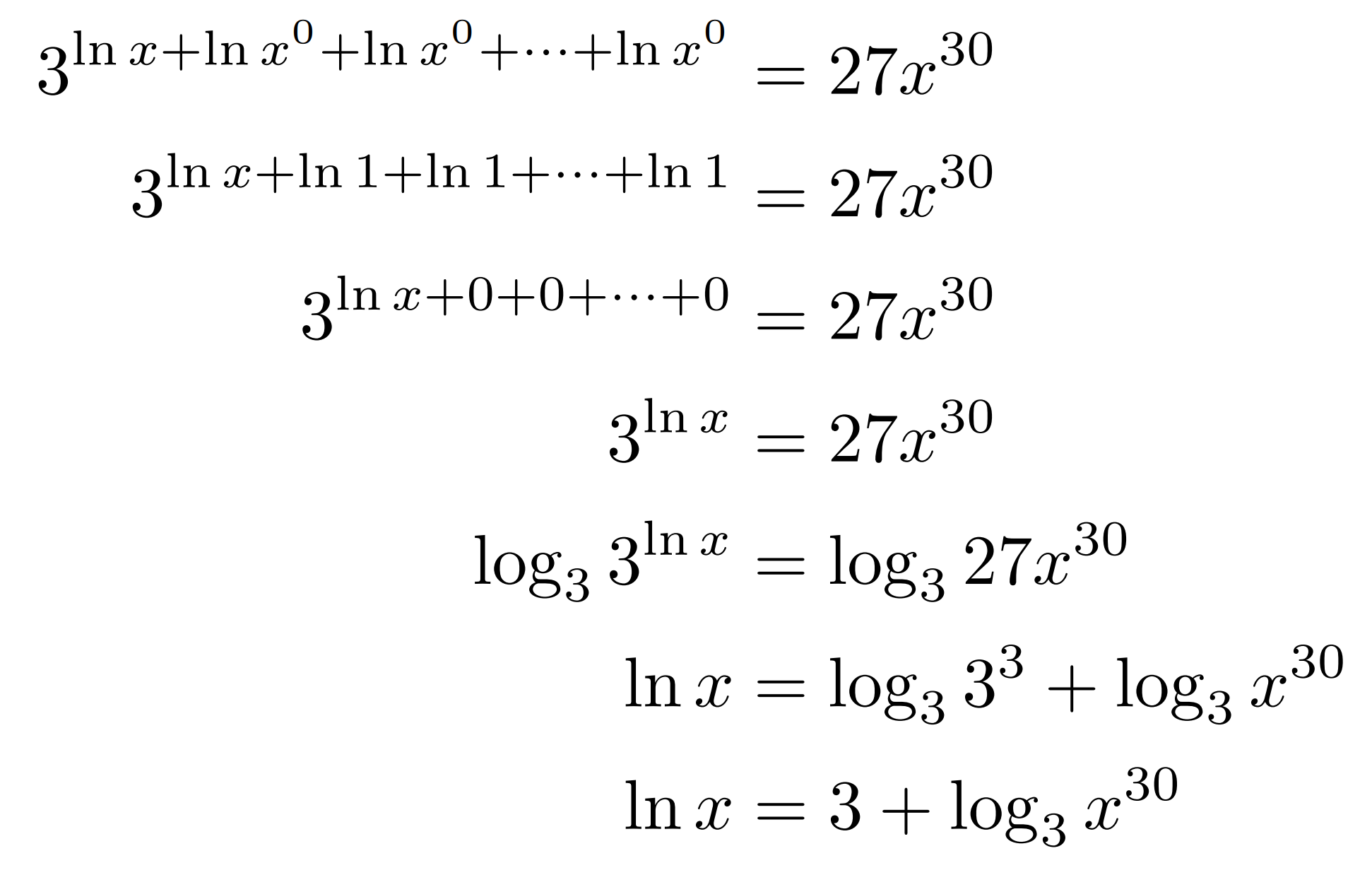
Again, the only part I feel confident about is the silly thing with ln(x0), but I think I went down the wrong path after that based on your answer. I could raise e to both sides to free the x on the left-hand side, but then I'm trapping the x on the right. I guess with both of them I'm struggling with the same thing: what to do when you have a mismatch between one side having a plain variable and the other having a variable inside a logarithm.
For problem two: I got to the answer by substituting a = 0.2^3 if you try that, you'll get two roots with the same things inside, leading you to compare only the root exponents
For problem 3: you're on the right track, just change the base of the logarithm on the left hand side by remembering ln(x) =log 3 (x)/log 3(e). From there, you just need to isolate all the x on one side, the log 3(e) on the other, and do 3 to the power of that to get just x on the left-hand side. Then you can use that property of logarithms on the right hand side to get all the log 3(e) in terms of ln(3) and simplify some more.
Thanks heaps—managed to get them both! It's good to know I wasn't too far off. I had a feeling I needed to do change of base but I wasn't exactly sure where I needed to go with it.
As the other comrade said, you've got the answers.
For (2)
- 2t2 + 7t -3 > 0
Or 2t2 - 7t -3 <0
We can find the roots and get 2t2 - 7t -3 as (t - 3) (2t - 1) < 0From this we can see that it's less than zero when t < 3, because the (t - 3) term becomes negative But if t < 1/2, both terms become negative and the equality is not valid.
Thank you. This shows both bounds. I had overlooked that and cancelled the (2t - 1) in a previous step.
So the answer would be 1/2 < ln x < 3
Thanks againFor (3) we have log3 x30 = 30 ln x / ln 3
Using the property loga X = logb X / logb aA question: How did you type of out the equations? LaTeX?
Is there any simple/foss app for it on Android?Thank you! They both seem so simple now that I've seen your explanation, but that's always how it is with these things; I'm sure we've all had the experience of nodding along to something in class only to be completely flummoxed by the same material when doing the homework later that day.
With (2), I had a feeling that I needed to factor, but I've never been that great at factoring quadratics when the second degree's coefficient isn't 1, and even if I had succeeded in factoring I don't think I would have remembered the logic to work through to get the final inequality. But now I understand thanks to your explanation!
solution for (2)
Show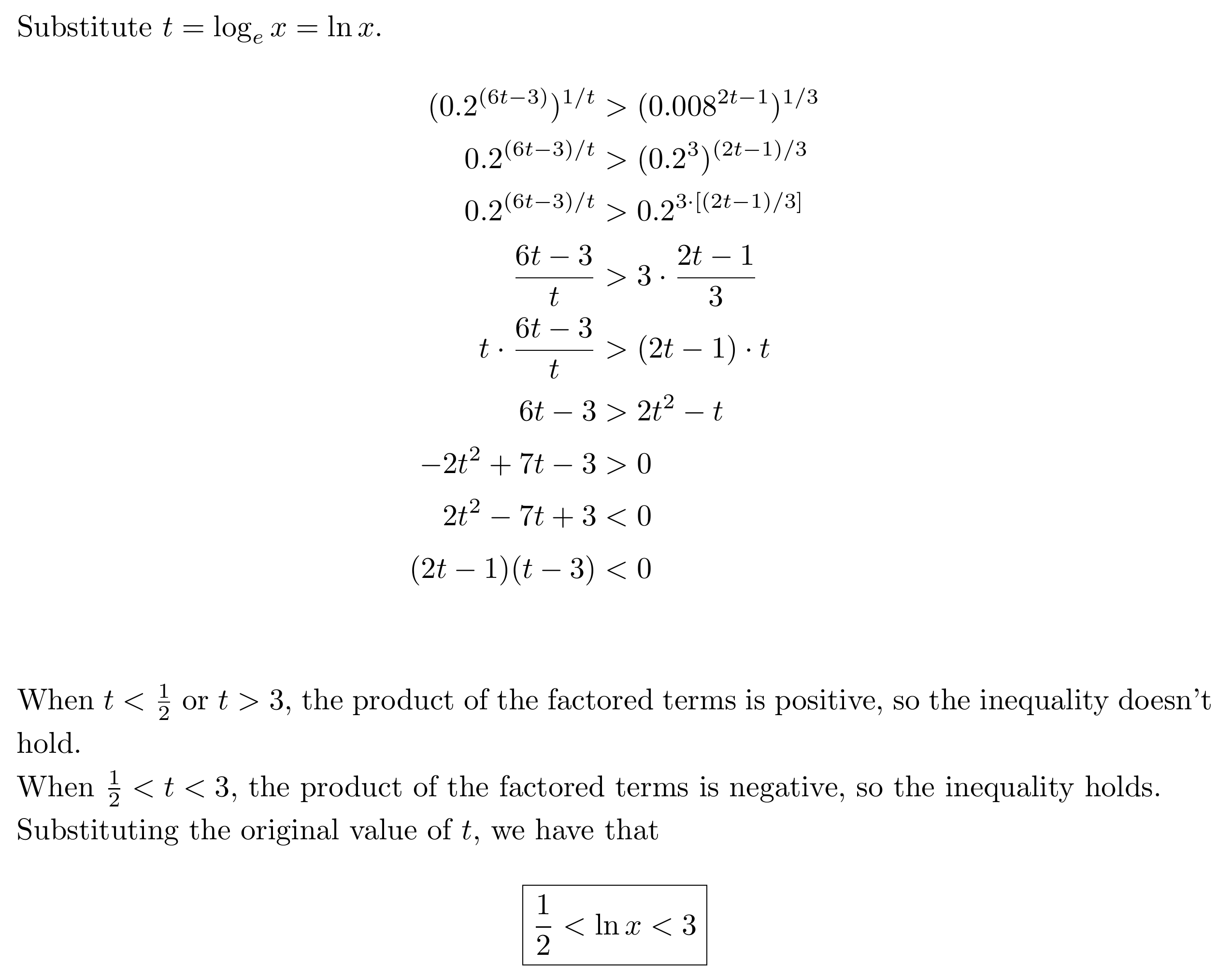
With (3), for some silly reason I thought that the rule with logaxb = b ⋅ loga x only applied when x = a (no idea why—obviously in that case you just have logaab = b) ...as I said, it's been a while since I did this stuff regularly. That was the key I needed to finish that one!
solution for (3)
Show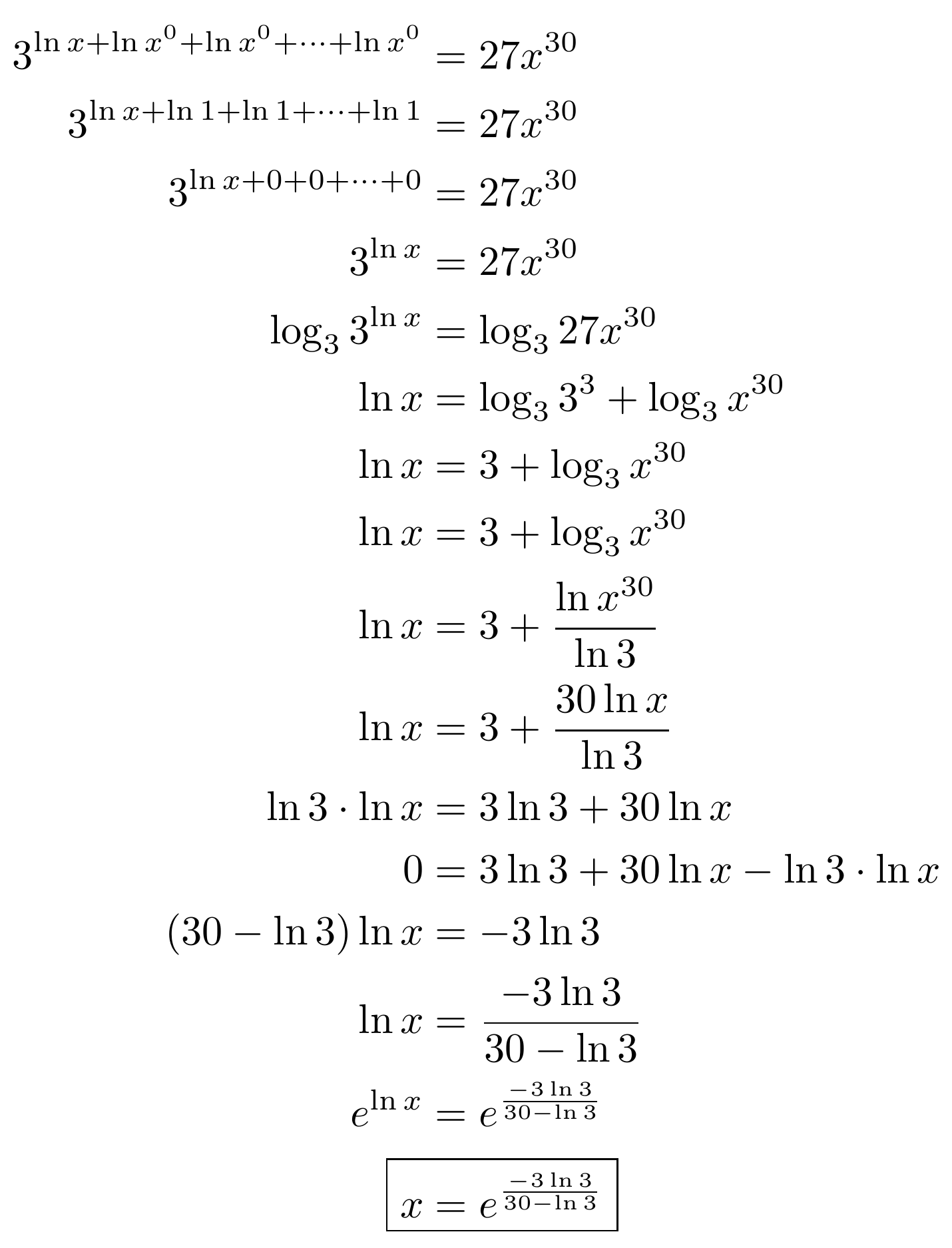
Yup, I used LaTeX! I'm not personally familiar with an app targeted at Android, but I used the web-based Overleaf, which is FOSS (AGPLv3). While the website I linked to does require the creation of a free account (which was all I ever needed for my simple undergraduate homework purposes--I think the premium features are only relevant for large projects/teams), you can even self-host it if you're into that sort of thing.
Incidentally, here's the LaTeX markup for the problems I worked (nothing fancy, just using the basic math packages with the main feature being the
\alignenvironment which allows me to center the equations around the = or >/< symbols).LaTeX markup
\documentclass{article} \usepackage{amsmath, amsthm, amssymb, amsfonts, mathtools} % ------------------------------------------------------------------------------ \begin{document} % (2) Substitute \(t = \log_{e}{x} = \ln{x}\). \begin{align*} (0.2^{(6t - 3)})^{1/t} &> (0.008^{2t - 1})^{1/3} \\ 0.2^{(6t - 3)/t} &> (0.2^{3})^{(2t - 1)/3} \\ 0.2^{(6t - 3)/t} &> 0.2^{3 \cdot [(2t - 1)/3]} \\ \frac{6t - 3}{t} &> 3 \cdot \frac{2t - 1}{3} \\ t \cdot \frac{6t - 3}{t} &> (2t - 1) \cdot t \\ 6t - 3 &> 2t^2 - t \\ -2t^{2} + 7t - 3 &> 0 \\ 2t^2 - 7t + 3 &< 0 \\ (2t - 1)(t - 3) &< 0 \\ \end{align*} When \(t < \frac{1}{2}\) or \(t > 3\), the product of the factored terms is positive, so the inequality doesn't hold.\\ When \(\frac{1}{2} < t < 3\), the product of the factored terms is negative, so the inequality holds.\\ Substituting the original value of \(t\), we have that \begin{equation*} \boxed{\frac{1}{2} < \ln{x} < 3} \end{equation*} %(3) \begin{align*} 3^{\ln{x} + \ln{x^0} + \ln{x^0} + \cdots + \ln{x^0}} &= 27x^{30} \\ 3^{\ln{x} + \ln{1} + \ln{1} + \cdots + \ln{1}} &= 27x^{30} \\ 3^{\ln{x} + 0 + 0 + \cdots + 0} &= 27x^{30} \\ 3^{\ln{x}} &= 27x^{30} \\ \log_3{3^{\ln{x}}} &= \log_3{27x^{30}} \\ \ln{x} &= \log_3{3^{3}} + \log_3{x^{30}} \\ \ln{x} &= 3 + \log_3{x^{30}} \\ \ln{x} &= 3 + \log_3{x^{30}} \\ \ln{x} &= 3 + \frac{\ln{x^{30}}}{\ln{3}} \\ \ln{x} &= 3 + \frac{30\ln{x}}{\ln{3}} \\ \ln{3} \cdot \ln{x} &= 3\ln{3} + 30\ln{x} \\ 0 &= 3\ln{3} + 30\ln{x} - \ln{3} \cdot \ln{x} \\ (30 - \ln{3})\ln{x} &= -3\ln{3} \\ \ln{x} &= \frac{-3\ln{3}}{30 - \ln{3}}\\ e^{\ln{x}} &= e^{\frac{-3\ln{3}}{30 - \ln{3}}}\\ \Aboxed{x &= e^{\frac{-3\ln{3}}{30 - \ln{3}}}} \end{align*} \end{document}Thank you. Since I have MS word, I sort-of make-do with it's equation editor,
Been interested in LaTeX for a while, so thanks for this.
No problem! I think it's definitely worth learning the basics, which I don't think are too bad, and are really plenty unless you have very specific needs or want to make complex diagrams. And regardless, the wizards on StackOverflow are always there to help.
By the way, I just noticed that there's an issue with the markup I posted due to a known Lemmy bug where ^ gets replaced with the
supHTML tag even in code blocks. I'll post a proper Pastebin or something when I get the chance!I thought that the LaTeX code used a syntax similar to html.
Thanks a lot.
No problem! Yeah, as you can see it's a bit different--basically, you've got commands that start with backslashes, which can have one or more arguments contained in curly brackets. The most important thing to understand is probably math mode which is what enables all the fancy mathematical typesetting.
Here's the Pastebin with the correct markup for my earlier comment:
https://pastebin.com/q3Sww7QM
-
i'm about to start fucking barking. i love maths and the idea that my one (1) skill would actually be useful in the USSR instead of just making me prime hiring material for banks is just

oh fuck this, just give me a submachine gun. Only math I need is figuring out how much is left in my mag
You have to understand many of us here are unfortunately American.
Damn you do need to remember some properties I completely forgot existed. Guess the soviets took their math classes very seriously.